Modes of heat transfer
- The main difference between thermodynamics and heat transfer analysis of a problem is that in thermodynamics we deal with system in equilibrium that is to bring a system from one equilibrium state to another equilibrium state how much heat is required is the main criteria in thermodynamics analysis.
- But in heat transfer analysis we evaluate at what rate this change of state occurs by calculating rate of heat transfer in joule per second or watt.
- Conduction is the mode of heat transfer which generally occurs in solid due to temperature difference associated with molecular lattice vibrational energy transfer and also by free electron transfer.
- The reason behind all electrically good conductors are also in general good conductor of heat is that the presence of abundant number of free electrons available in the outermost orbit example all metals.
- Notable exception to the above statement is diamond because the conductivity of diamond is 2300 w / m k. The highest thermal conductivity of diamond is due to its perfect crystalline molecular lattice arrangement of structure.
- Thermal conductivity is a property of material which tells about the ability of the material to allow the heat energy to get conducted through the material more rapidly or quickly.
- Insulators have a very low value of thermal conductivity thereby can prevent or reduce the heat transfer rate.
- Example asbestos 0.2 w / m k ,refractory brick 0.9 w / m k.
- Heat conduction can occur through gases by molecular momentum transfer when high velocity high temperature molecules collide with the low velocity low temperature molecules but in general ,gases are very bad conductors of heat.
- Example conductivity of air at room temperature is 0.026 w / m k. As the temperature of the gases increase their by thermal conductivity also increases because at higher temperature greater molecular activity of gas molecules may result in more number of collisions per unit time and hence momentum transfer rate.
- Lighter gases have more thermal conductivity.
- Liquids are better conductor of heat than gases.
- Among all liquids Mercury has highest thermal conductivity.
- Convection is a mode of heat transfer with generally occurs between a solid surface and the surrounding fluid due to temperature difference associated with the macroscopic bulk motion of fluid transport in thermal energy in the form of enthalpy.
- In case of forced convection heat transfer this motion of the fluid is provided by an external agency like a fan or a blower or a pump where is in free convection heat transfer the motion of the fluid occurs naturally due to byouant forces ,arising out of density changes of fluid because of its temperature changes.
- Prevost's theory : all bodies at all temperature emit thermal radiation except the body at zero kelvin.
- The rate of emission is directly proportional to T^4.
- Radiation is the mode of heat transfer which does not require any material medium for its propagation and hence occurs by electromagnetic wave propagation travelling with the speed of light. The radiation mode of heat transfer completely predominates over conduction and convection particularly when the temperature difference is sufficiently large.
- Example the mode of heat transfer between hot flue gases and refractory brick walls in a large pulverised fuel fired power boiler is predominantly by thermal radiation ,reason: because of large temperature difference.
Governing laws of heat transfer
Fourier's law of conduction
- The law states that the rate of heat transfer by conduction along a given direction is directly proportional to the temperature gradient along the direction and is also directly proportional to the area of heat transfer line perpendicular to the direction of heat transfer.
- The law states that the rate of heat transfer by convection between a solid body and the surrounding fluid is directly proportional to the temperature difference between them and it is also directly proportional to the area of contact of area of exposure between them.
- unlike thermal conductivity ,convective heat transfer coefficient is not a property of the material but it depends upon some of the thermophysical properties of the fluid like density, viscosity, specific heat and thermal conductivity.
- Practical ranges of convective heat transfer coefficient
- The law state that radiation energy emitted from the surface of a black body per unit time per unit area is directly proportional to the fourth power of the absolute temperature of the black body.
- Assume :steady state heat transfer condition. One dimensional heat conduction. No heat generation in slab and constant thermal conductivity.
- Lesser the conductivity of slab and higher the thickness of the slab more the conduction thermal resistance offered by the slab and lesser will be heat current.
- Assume :steady state one dimensional conduction heat transfer through composite slab.
- For steady state one dimensional conduction heat transfer thermal circuit
- Assume: steady-state one-dimensional conduction convection heat transfer between hot gases and the ambient colder fluid through composite slab
- Thermal circuit
- overall coefficient defining :overall heat transfer coefficient U as the parameter which takes into account all the modes of heat transfer into a single entity that is from the equation q = UA dT
- If U value is more it means the total thermal resistance against the heat flow is lesser thus heat current will be more
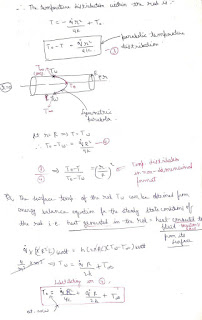
- Since temperature gradients are existing along the radial direction ,heat must conduct radially outwards from the inner cylindrical surface at T1 to outer cylindrical surface at T2
- assume steady-state one-dimensional conduction heat transfer with constant thermal conductivity. Unlike in case of slabs here the area of conduction heat transfer changes in the direction of heat flow. At any radius r area of heat conduction heat transfer .
- If the thickness of cylinder is very small and if the conductivity of cylinder is very high the above conduction thermal resistance almost become zero.
- Since temperature gradients are existing along the radial direction ,heat must conduct radially outward from the inner speripheral surface at T1 to outer circle at T2. Like in case of cylindrical heat transfer here also the area of heat conduction heat transfer changes in the direction of heat flow.
- Assume steady state one dimensional radiation conduction heat transfer.
Note:
- Two different geometry are supposed to be thermally equivalent in the heat transfer sense only if both of them are having the same total thickness and over the same thermal resistance.
- Assume steady state one dimensional conduction convection heat transfer between the hot gases flowing inside and the ambient colder fluid.
- Thermal circuit
- But in heat exchanger analysis weather log mean temperature difference method or effectiveness NTU method ,you can be calculated as:
- For sufficiently thin wires putting the insulation around the wire may result in increase of heat transfer rate instead of decreasing it.
- Consider a solid wire of radius Ri inside which heat is been generated by passing electric power , let an insulation having a thermal conductivity k1 is being wrapped around a wire up to the radius R0. The heat generated inside a wire due to passage of current is radially conducted through the insulation from the surface of the wire and then from the surface of the insulation heat is convected to the ambient air with the convective heat transfer coefficient of h watt/meter square k.

- Let ti be the surface temperature of the wire under steady state heat transfer condition.
- For sufficiently thin cables whose radius is less than critical radius of insulation putting the insulation around the wire will result in increase of heat transfer rate instead of decreasing it. This happens so because initially when more and more insulation is being wrapped around the wire there is a rapid decrease in convection thermal resistance as compared to little increase of conduction thermal resistance the overall effect being decrease in total thermal resistance and hence increase of heat transfer rate. This continues to happen up to a critical radius of insulation beyond which any further insulation will decrease the heat transfer rate.
- Electrical power transmission cables :Insulation is provided around the electrical power transmission cable to increase the heat transfer rate between the cables and the ambient so that the temperature of the cable can be maintained lo thereby its electric resistance can be maintained lo the transmitting more electric power.
- Spherical electronic devices
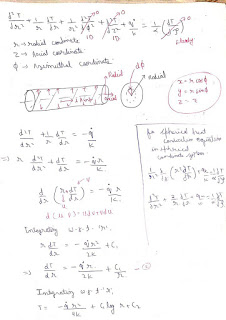
Generalized heat conduction equation (3D steady or unsteady , with or without heat generation)
- Heat can be generated inside a solid body either by supplying electric power or by exothermic chemical reaction or by thermonuclear fusion.
- Consider a differential element of the slab thickness dX as shown in the figure.
- If Qx is the heat conducted into the element and qx + dX is heat conducted out of the element.
- Writing the energy balance similarly for all the three directional heat conduction that are occurring along X ,y and z direction simultaneously we get ,
- Thermophysical property of material as the ratio between thermal conductivity of the material and its thermal capacity that is thermal diffusivity , thermal diffusivity of a medium or a material signifies the ability of the material to allow the heat energy to get diffused or pass through the medium more rapidly or quickly.
- Higher the conductivity of material and lesser its heat capacity or heat storage ability, more the value of thermal diffusivity of material.
- Objective to get temperature distribution within the slab.
- Assumptions steady state heat transfer condition.
- To maintain the steady state condition of the slab while generating heat all the heat generated in the slab must be convected to a fluid either from one side of the slab or from both the sides.
- One dimensional heat conduction only, uniform heat generation rate and constant thermal conductivity of material.
- C1 and c2 are the constant of integration that are to be obtained from boundary condition.
- One special boundary condition that is both side of the slab subjected to the same temperature.
- This can be possible only both sides of the slab are exposed to the same fluid at the same temperature with the same convective heat transfer coefficient.
- To satisfy this boundary conditions C1 must be zero.
- C1 will be zero only if both sides are at same temperature. In case if both the sides of the cylinder at different temperature then C1 will not be zero which means that we will not get maximum temperature at the midplane of the slab.
- Objective to get temperature distribution within the rod.
- Assume steady state heat transfer condition. To maintain the steady state condition of the rod while generating heat all the heat generated in the rod must be convected to a fluid surrounding the rod at ambient temperature with the convective heat transfer coefficient of h w /m square k.
- One dimensional heat conduction.
- Uniform heat generation rate and constant thermal conductivity of material.
- The generalized heat conduction equation in a cylindrical co-ordinate system is given by
- If heat transfer rate are very high and if h values are low that T0 values may increase enormously which may finally result in melting of the rod itself and the melting begins at the axis of the rod .

















































0 Comments